Fractions / Percentages – Definitions of Terms

Denominator: The bottom number in a fraction. Example: The denominator of 2/3 is 3.

Equivalent Fractions: Two fractions are equivalent if one can be transformed to the other by simply multiplying both numerator and denominator by the same number. Example: 1/2 is equivalent to 4/8, because if numerator and denominator of 1/2 is multiplied by 4, we get 4/8.

Factor: A component of a multiplication product. For example, 2 and 3 are factors of 6, since 6 = 2 x 3.

Fraction: A number, also called a rational number, which is a ratio of two integers, consisting of a numerator and a denominator. 3/4 is a fraction with the numerator being 3 and the denominator being 4.

Greatest Common Factor (Divisor): The largest number which evenly divides two or more numbers. The greatest common factor (or the greatest common divisor) of 20 and 24, for example, is 4. Even though 2 evenly divides both numbers, it’s smaller than 4, so 4 is the greatest common factor.

Inverse: The inverse of a number x is a number which when multiplied by x yields 1 as the product. Example: The inverse of 2 is 1/2, because 2 × 1/2 = 1. Also, the inverse of 3/4 is 4/3; the inverse of 1 is 1; and the inverse of -1 is -1. The inverse is often called the reciprocal because the inverse of a fraction is the fraction inverted, as in 3/4 is the inverse of 4/3, and 4/3 is the inverse of 3/4. The only number without an inverse is 0, because division by 0 is undefined, so 0 has no reciprocal.

Improper Fraction: A fraction whose numerator is larger than its denominator. Example: 3/2

Least Common Multiple (lcm): The smallest number which is a multiple of two or more numbers. For example, the least common multiple of 6 and 10 is 30, since even though 60 is a multiple of both numbers, 30 is also and is smaller than 60.

Numerator: The top number in a fraction. Example: In the fraction 3/4, 3 is the numerator.

Percentage: A fraction expressed in 100th’s, in which only the numerator is written, and the denominator is assumed to be 100. Example: 50 percent (written 50%) actually means 50/100.

Prime Factorization: The representation of a positive integer as a product of powers of primes (a p-p-p!). For example, the prime factorization of 180 is

\(\begin{align} 2^2 \times 3^2 \times 5\end{align}\)

since the only prime numbers that divide 180 are 2 (twice), 3 (twice), and 5.

Prime Number: An integer greater than 1 which can only be evenly divided by itself or by 1. Examples: 2, 3, 5, 17, 43.

Proportion: A statement of equality between two fractions or ratios. Thus, a proportion problem might be x/2 = 8/4, for which x = 4.

Ratio: A fractional relationship between 2 numbers. Thus, the ratio of a to b is a/b. It can also be shown as a:b.

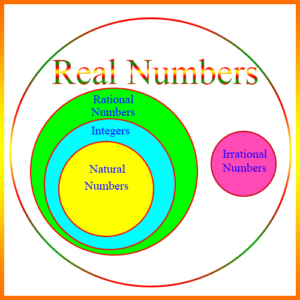
Rational Number: A number which is a fraction or “ratio” between two integers. 2/5, 25/8, -4/3, and 0 are all rational numbers. Every integer is a rational number. Rational numbers are also considered real numbers. The figure shows rational numbers as a subset of real numbers and containing integers and natural numbers as subsets.

Reciprocal: The inverse of a number. If a number is multiplied by its reciprocal, then the product is 1. Examples: The reciprocal of 2/3 is 3/2, so 2/3 × 3/2 = 1. The reciprocal of 5 is 1/5, so 5 × 1/5 = 1.